What are Horizontal Stretches and Shrinks?

Horizontal stretches and shrinks, respectively, horizontally pull the base graph, or push it together, while leaving the y-intercept unchanged to anchor the graph.

A horizontal stretch or shrink by a factor of 1/k means that the point (x, y) on the graph of f(x) is transformed to the point (x/k, y) on the graph of g(x).

Examples of Horizontal Stretches and Shrinks

Consider the following base functions,

(1) f (x) = x² - 3,

(2) g(x) = cos (x).

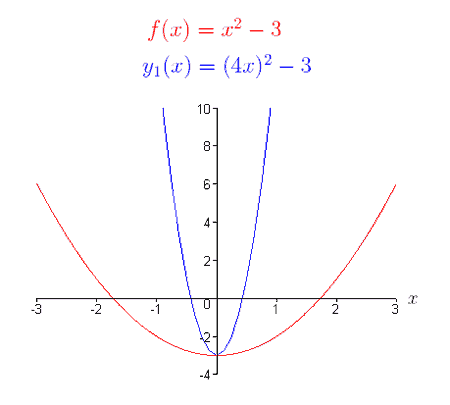
The graphical representation of function (1), f (x), is a parabola. What do you suppose the graph of

y₁(x) = f (4x)

looks like? Using the definition of f (x), we can write y₁(x) as,

y₁(x) = f (4x) = (4x)² - 3 = 16x²-3.

Based on the definition of horizontal shrink, the graph of y₁(x) should look like the graph of
f (x), shrunk horizontally by a factor of 1/4. Take a look at the graphs of f (x) and y₁(x).

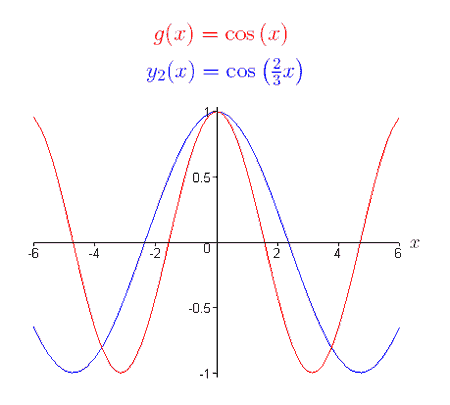
Function (2), g (x), is a cosine function. What would the graph of

y₂(x) = g(2/3x)

look like? Using our knowledge of horizontal stretches, the graph of y₂(x) should look like the base graph g(x) stretched horizontally by a factor of 3/2. To check this, we can write y₂(x) as,

y₂(x) = g(2/3x) = cos (2/3x),

construct a table of values, and plot the graph of the new function. As you can see, the graph of y₂(x) is in fact the base graph g(x) stretched horizontally by a factor of 3/2.

*****

In the next section, we will explore reflections.

Reflections