- Your homework: graded pp. 136--, #17, 47, 51
- #17: We usually prefer the answer in the form of a quotient (rather than as a sum of three terms). But different strategies give different results (as does Mathematica).
- #47: some of you don't feel compelled to answer the
question "Explain..." I mean you to! In this case it's about symmetry.
$f$ is not quite odd -- but it's only off by a constant. So its derivative will be even, and the second derivative will be odd, and so on.
- Show work! Especially when the answers are in the back....
- Derivative of tangent
- Fourth derivative of sine, cosine
- #7, p. 146
- #26
- #35
- #49 and 50
- The chain rule is so critically important because of the
importance of compositions of functions: for
$F(x) = f(g(x))$,
$F^\prime(x) = f^\prime(g(x))g^\prime(x)$ I personally think this to myself: "f prime of stuff times the derivative of the stuff"; or "f prime of stuff times stuff prime."
:
$F^\prime(x) = f^\prime(stuff)stuff^\prime$ You can see that the rule is fairly simple, once you've identified the composition -- that is, once you've torn apart $F$ to find $f$ and $g$.
We took a look at a file from my pre-calc class to review compositions. Remember?
- The difficulty is often identifying the composition. We'll try
some easy ones first, then get to some ugly ones.
Before we do that, however, I'd like to show how to derive the chain rule, using the limit definition of the derivative. Everything comes from that! I'll need to use a result from p. 153, where the chain rule is proved in our text.
Examples:
- #9, p. 154
- #16
- #29
- #42
- #77
- A Chain Rule tutorial
- A sample problem involving compositions: model the seasonal variation in Keeling's CO2 data:
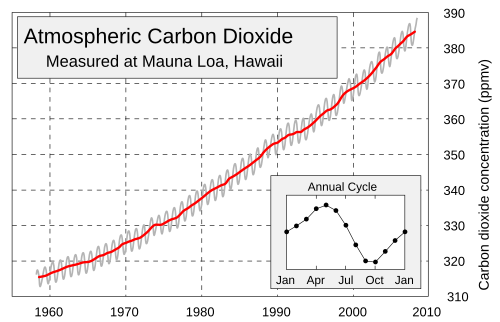
If time were measured in years from January, would
$V(t)=A\sin(2\pi{t})$ be a good model? What would be a good choice for the parameter $A$?