- Reminder: turn off your rectangles.
- Your quizzes are returned, as is your first homework.
- quizzes: you're getting the definition of function down, at any rate! Some of you still don't know, however....
- homework: randomly chose
2 and 7 to grade.
- Each is graded out of two, so your
assignment is out of 4.
- Answer each question completely. On problem 2, for example, it asked "What are your conclusions?"
-
Don't forget units. Units can be very helpful. For example, in problem 2 you're looking for heart rate (beats per minute). This suggests the ratio (difference in heart beats)/(elapsed time). Some of you had that reversed.
- You need to show work, especially on the odd problems where the answers are printed in the back....
- Each is graded out of two, so your
assignment is out of 4.
- You have an assignment for section 1.5 due Wednesday.
- #18, p. 60
- #26, p. 60
- #35, p. 61
- #42, p. 61
Questions about anything at all (including homework for 1.5), before we move along?
- Limit laws:
- We start with two especially trivial special limits:
- Constant rule:
- Identity rule:
What can we do with those? We need more horsepower....
- Constant rule:
- Sum law: "the limit of a sum is the sum of the limits"
So what is this limit:
This sum (and many other operations) satisfy the same pattern:
"The limit of a STUFF is the STUFF of the limits." So, in particular,
"The limit of a sum is the sum of the limits." - Difference law
- Product law
- Quotient law (slight caveat here -- no zero denominators)
- Power law
- Root law -- again, a caveat: why can't we compute
\[ \lim_{x\to{0}}\sqrt{x} \]
Because the function isn't even defined as x approaches 0 from the left.
- Putting these laws together
- Now that we've got the sum and product and constant multiple rules, what can we deduce?
- "Affine" rule, for one:
\[ \lim_{x\to{a}}(mx+b)=ma+b \] - Then we can proceed to deduce that all polynomials have limits, and
furthermore that
This is the conclusion of Exercise #55, p. 71, which says that polynomials are continuous, which we'll consider more in section 1.8.
Exercise #56 says it's also true for rational functions, for every element of their domain.
- Other Laws
- The squeeze theorem
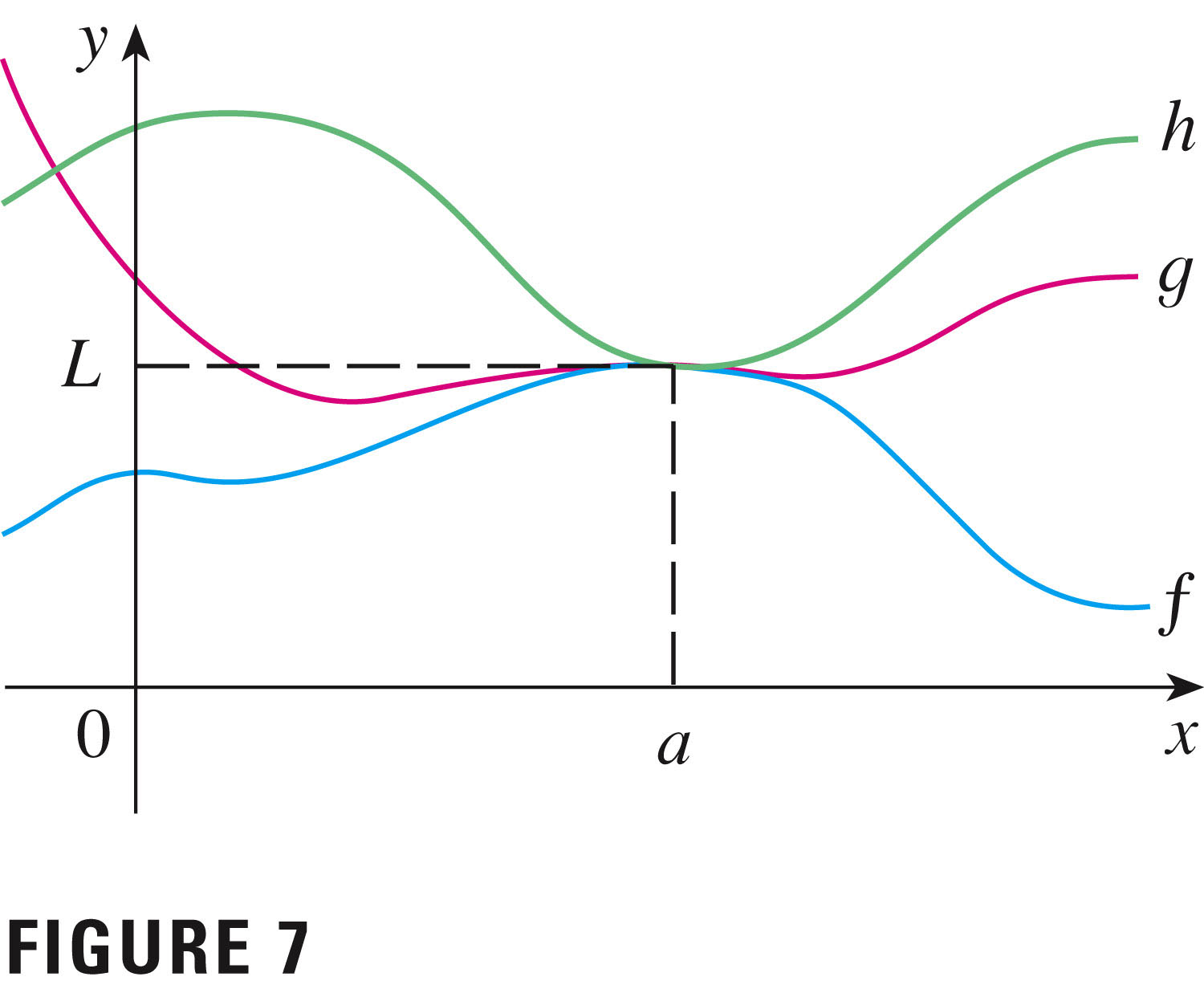
- The squeeze theorem
- Some Examples
- Additional examples:
- #63, p. 71
- #59, p. 71 (squeeze it!)
- We start with two especially trivial special limits: