Electronic Supplement to The College Mathematics Journal

Vol. 29, No.2, March 1998

Order of Integration and a Minimal Surface.

Thomas Hern, Cliff Long, and Andy Long

We reproduce here the figures from the article in color, and in larger size, and add stereo views, and a movie sequence, which might aid the reader.

The intent of this article was twofold:


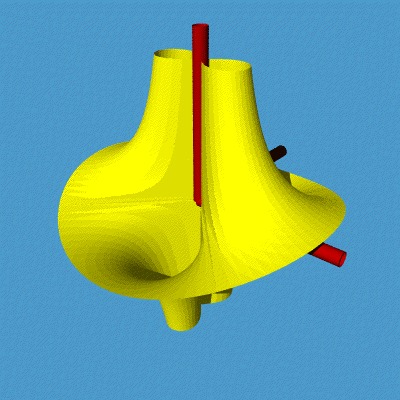
Figure 1
[Zoom]

First of all to examine visually the function
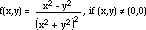which shows that the order of integration is important when trying to evaluate a double integral of a function when using iterated integrals. We will call this surface the Fubini surface, after Fubini's Theorem.

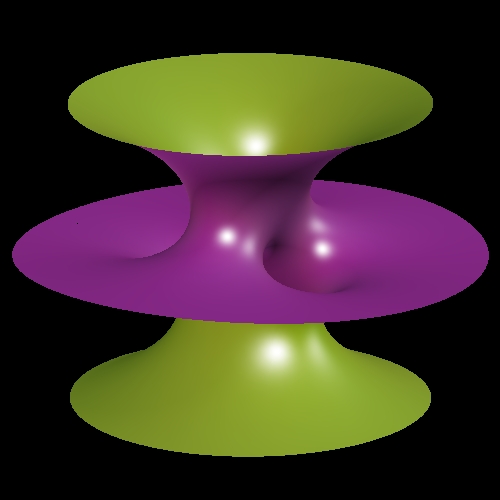
Figure 2
[Zoom]
And, secondly, to utilize the Fubini surface as a tool in understanding a more complicated surface - a minimal surface known as the Costa Surface and visually studied by David and James Hoffman. Shown here.

A new exhibit, Beyond Numbers, at the Maryland Science Center in Baltimore contains a nice display of this surface.


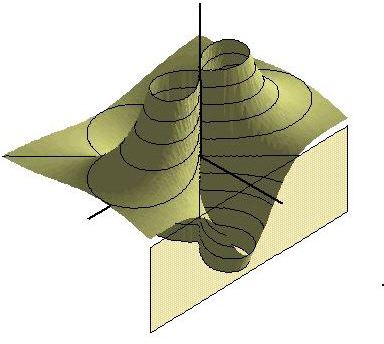
Figure 3
[Zoom]

Iterated integrals leading to different results for the Fubini surface.

This view of the Fubini surface sliced by the plane y = .5 shows that the integral with respect to x will be negative for all values of y. (Here is a graph of this cross section to make it clearer.) However, the correponding view with a plane in the other direction is this curve inverted, which means a positive value for the integral with respect to y for all values of x. And thus the second integrations will then be of opposite signs. Actually the two iterated integrals on [-1,1] x [-1,1] are -¹ and ¹. Hence, the double integral fails to exist.

We have drawn the level curves on the surface. The key observation here is that the level curves are nested lemniscates (except at (0,0), and since all level curves converge to the origin, every real number is taken on in every neighborhood. So there cannot be a limit there. The function fails to be continuous at (0,0), hence Fubini's Theorem does not apply.

The transition between these two surfaces

We were rather surprized to notice a strong visual connection between the Fubini and Costa surfaces, i.e., Fubini's is topologically equivalent to a pinched Costa surface: the point of the paper. This is easily seen via the following sequence of intermediate views of a transition between the surfaces, and even easier when these are viewed in a movie format. It is most effective if you loop the movie back and forth in the viewer.


Figure 4
[Zoom]

An MPEG movie of this transition.

An animated GIF.

A Quicktime movie.

For those interested in stereo viewing

The following are stereo views of the Fubini surface. There are three views across the picture. The left two views are a stereo pair for those viewers who prefer cross eyed viewing, and the right two views are a stereo pair for those who prefer straight ahead ("wall-eyed") viewing. It is quite possible to see depth inherent in the right pair without the aid of a viewer. (Simply get each eye looking at its own view. A distance of about 15 inches is best and some people find the placement of a 3 X 5 card on edge between the eye views to be helpful. Relax the eye convergence much like looking at a random dot stereogram. A critical restriction for stereo viewing is to keep your eye level parallel to the picture horizontal. Alternately closing the left and right eyes sometimes helps in homing in on the depth cue.)

Figure 3 was done with Maple, Figure 2 was provided by James Hoffman, Scientific Graphics Project, MSRI. All others were raytraced with Rayshade.

Thomas Hern (hern@math.bgsu.edu), Cliff Long (long@math.bgsu.edu)
Bowling Green State University

Andy Long (ael@maya.medctr.luc.edu)
Fulbright Scholar in Benin, West Africa.